

Develop spatial imagination
0.00 $
0 item(s)
Platonic solids. Platonic polyhedra. How to make pdf template
This group of five geometric solids was given the name of the ancient Greek scientist Plato. Mathematicians call these five polyhedra regular, but we most often call them the Platonic solids in ordinary speech.
First, let us identify these five geometric objects — or, in the terminology of mathematicians, geometric solids.
First of all, why are these five geometric solids called regular polyhedra?
This is quite easy to remember. The sides of regular polyhedra are regular polygons. In turn, regular polygons are those in which all sides are equal (e.g., triangle, square) and have equal angles between adjacent sides. This is why they are called "regular".
What is the connection with Plato?
The ancient Greek scientist Plato was most likely not related to discovering these remarkable polyhedrons, but Plato had another talent. In the modern world, Plato could be called the popularizer of regular polyhedra. The greatest contribution Plato made is that he told people about the existence of regular polyhedra.
If this is all he had done, however, the majority would have quickly forgotten about them. But Plato endowed these seemingly simple objects with incredible strength and mystical meaning and brought them to the forefront of his teaching.
In an attempt to explain the nature of everything that exists, Plato considered five regular polyhedra to be fundamental principles for the structure of each of the elements:
- fire correlated with the tetrahedron;
- air correlated with the octahedron;
- earth correlated with the hexahedron;
- water correlated with icosahedron;
- and the dodecahedron corresponded to the Universe.
It was the power of Plato's philosophy and mystical postulates that were entrenched in ordinary people's minds, which inextricably linked by these polyhedra with Plato's ideas. And, at some point, people began to talk about five regular polyhedra as Platonic solids.
Popular
Young British designer, Richard Sweeney, creates amazing paper sculptures. Interestingly, regular...
Quite often, a question about the practical use of paper models pops up. What is the point of...
Is it possible to conduct additional school classes in geometry collecting models of polyhedra? Of...
Summer is the time you want to spend outdoors. At the desk, the children will sit down in...
The new Atom Browser creators decided to choose the image of a polyhedron or an icosahedron for...
One of the most famous magazines in Russia - popularizers of science with 115 years of history-...
Is it possible to make up an icosahedron using more simple polyhedra?...
















 Three triangular faces converges at the same vertex of the tetrahedron. The three-dimensional angle between the three faces (solid angle of a tetrahedron at the vertex) is Ω = 0,55.
Three triangular faces converges at the same vertex of the tetrahedron. The three-dimensional angle between the three faces (solid angle of a tetrahedron at the vertex) is Ω = 0,55.

 Four triangular faces converges at the same vertex of the octahedron. The three-dimensional angle between the four faces (solid angle of an octahedron at the vertex) is Ω = 1,36.
Four triangular faces converges at the same vertex of the octahedron. The three-dimensional angle between the four faces (solid angle of an octahedron at the vertex) is Ω = 1,36.

 Three quadrangles faces converges at the same vertex of the cube. The three-dimensional angle between the three faces (solid angle of a cube at the vertex) is Ω = 1,57.
Three quadrangles faces converges at the same vertex of the cube. The three-dimensional angle between the three faces (solid angle of a cube at the vertex) is Ω = 1,57.
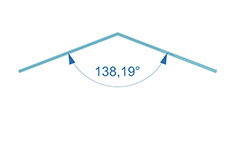
 Five triangular faces converges at the same vertex of the icosahedron. The three-dimensional angle between the five faces (solid angle of an icosahedron at the vertex) is Ω = 2,63.
Five triangular faces converges at the same vertex of the icosahedron. The three-dimensional angle between the five faces (solid angle of an icosahedron at the vertex) is Ω = 2,63.

 Three pentagonal faces converges at the same vertex of the dodecahedron. The three-dimensional angle between the three faces (solid angle of a dodecahedron at the vertex) is Ω = 2,96.
Three pentagonal faces converges at the same vertex of the dodecahedron. The three-dimensional angle between the three faces (solid angle of a dodecahedron at the vertex) is Ω = 2,96.





