This little "joke" task will keep your kids (students) busy for a while!

What plastic tetrahedron* needs to be melted to cast a cube with an edge length of 50 mm from this plastic?

An interesting feature is that our brain is used to the perception of volume through a container in the form of a cube. But it is difficult to imagine a comparable volume in the form of a tetrahedron.

* the tetrahedron is a triangular pyramid, in which all three sides and base are equal to each other and represent a regular triangle.

In More Detail, the Task Can Be Formulated As Follows:

We have 4 tetrahedrons. All 4 tetrahedrons are made of the same plastic. And filled with this plastic. We can find out (check) the value of the edge length by attaching an ordinary ruler.

Question: By melting which tetrahedron can we get a cube with an edge equal to 50 mm?

We assume that the entire process of melting and transformation of the plastic tetrahedron occurs without losses. That is, the plastic from which the tetrahedron was made goes into a cube.

What We Are Going to Make.

To "warm-up" the interest of schoolchildren in solving this problem, we will make four tetrahedrons and one cube from plastic shape nets.

We need:

- 5 sheets of PVC plastic film (0.25 mm thick). A4 format;

- knife (+ scissors);

- cutting mat.

Print out shape nets for cube and four tetrahedrons.

If you can't find PVC plastic film, you can use paper. Even plain white office printer paper will work.

download Cube shape net for assembly without glue
download Tetrahedron 1 shape net for assembly without glue
download Tetrahedron 2 shape net for assembly without glue
download Tetrahedron 3 shape net for assembly without glue
download Tetrahedron 4 shape net for assembly without glue

Making a Plastic Cube.

Secure the printed cube shape net to the PVC plastic film.

I suggest doing this with regular glue.

In the designated places, holes should be made with a clerical knife.

The fold lines must be slightly pressed.

This can be done with a pen and ruler.

Then the cube shape net must be cut along the contour. From my point of view, it is more convenient to do this with scissors.

Remove the auxiliary sheet of paper.

Remaining glue from the plastic part can be removed with a cotton swab dipped in water.

We bend the cube shape net.

We connect the shape net into a single geometric shape.

Making a Plastic Tetrahedron.

The operation of creating a tetrahedron is completely analogous to creating a cube.

Secure the printed tetrahedron shape net to the PVC plastic film.

I suggest doing this with regular glue.

In the designated places, holes should be made with a clerical knife.

The fold lines must be slightly pressed.

This can be done with a pen and ruler.

Then the cube shape net must be cut along the contour. From my point of view, it is more convenient to do this with scissors.

Remove the auxiliary sheet of paper.

Remaining glue from the plastic part can be removed with a cotton swab dipped in water.

We bend the tetrahedron shape net.

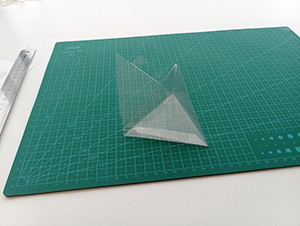
We connect the shape net into a single geometric shape.

We repeat the operation for the remaining shape nets.

The solution to the Task:

This task is easy to solve if we had these items made of solid plastic (filled with plastic inside).

We would just weigh them. And we will find a tetrahedron with a suitable mass equal to the mass of a cube!

But we will have empty plastic tetrahedrons and a cube. And weighing it won't help us.

But if you want the students to get a little confused, you can put a scale nearby.

So what do we know?

Only the length of the edge of each geometric solid.

The length of the edge can be measured with a ruler.

What do we need to find?

Of course, the volume of each solid! In other words, how much "liquid" plastic can fit inside.

That is, to solve the problem, we need to find a tetrahedron with a volume equal to the volume of a cube.

Hopefully, Does everyone remember how to find the volume of a cube? Multiply the length by the width and by the height (50x50x50).

But only a few people remember the formula - how to find the volume of a tetrahedron!

We'll have to look at Wikipedia.

Small digression.

But even here the comic task can be extended! After all, we don't have to say that we have four tetrahedrons. We can say that these are triangular pyramids. And triangular pyramids can have different heights and remain a triangular pyramid. Then, for the solution, from all the pyramids it will be necessary to select the one with all sides of equilateral triangles. And find a formula to calculate the volume of this pyramid.

The task solution in the picture.

Answer: melting a plastic tetrahedron with an edge length of 102 mm, we can cast a cube with an edge length of 50 mm.

This is the second-largest tetrahedron (out of the original four).

© polyhedr.com 23/11/2020